Section 4.3 Richard Rado
There are almost as many types of mathematicians as there are types of human being. Among them are technicians, there are artists, there are poets, there are dreamers, men of affairs, and many more. — Richard Rado
Who was Richard Rado? A mathematician who earned two Ph.D.s: in 1933 from the University of Berlin under Issai Schur, and in 1935 from the University of Cambridge under Godfrey Harold Hardy.
| Convergence of sequences and series | Inequalities | Ramsey theory |
| Geometry and measure theory | Number theory | Graph theory |
Birth and Death.Richard Rado was born on 28 April 1906 in Berlin, Germany, and died on 23 December 1989 in Henley-on-Thames, Oxfordshire, England.
World in 1906
World in 1989
The first radio set advertised
The first woman elected to American Society of Civil Engineers
The first animated cartoon copyrighted
Albert Einstein introduces his Theory of Relativity
Mahatma Gandhi coins the term “Satyagraha”
Alberta adopts Mountain Standard Time
SOS adopted as warning signal
Lee de Forest patents a 3-diode amplification valve
Richard's Family. Richard was born in Berlin. He was the second son of Leopold Rado, who was a Hungarian from Budapest. As a young man he had to choose between being a concert pianist or a mathematician. He chose to become a mathematician in the belief that he could continue with music as a hobby, but that he could never treat mathematics in that way.
In 1933 he married Luise Zadek, whom he had met when he needed a partner to play piano duets. They had one son, Peter Rado, born in 1943.
Rado and his wife had a double partnership: she went with him to mathematical conferences and meetings and kept contact with his mathematical friends, he was an accomplished pianist and she was a singer of professional standard. They gave many recitals both public and private, often having musical evenings in their home in Reading. Rado was the kindest and gentlest of men. (Source MacTutor.)
Paul Erdős about Richard Rado:
I first became aware of Richard Rado's existence in 1933 when his important paper Studien zur Kombinatorik appeared. I thought a great deal about the many fascinating and deep unsolved problems stated in this paper but I never succeeded to obtain any significant results here (…) Our joint work extends to more than 50 years; we wrote 18 joint papers (…) Our most important work in undoubtedly in set theory and, in particular, the creation of the partition calculus. The term partition calculus is, of course, due to Rado. Without him, I often would have been content in stating only special cases. (Source My joint work with Richard Rado).
Canadian Connection.
Canadian Commonwealth Fellow, University of Waterloo, 1971-1972
Visiting Professor, University of Calgary, 1973-1974
Hon. D. Mathematics, University of Waterloo, 1986
Rado's Work - Two Examples.
Example 4.3.4.
Partition Calculus:
In Erdős and Rado's words:
The investigation centres round what we call partition relations connecting given cardinal numbers or order types and in each given case the problem arises of deciding whether a particular partition relation is true or false. It appears that a large number of seemingly unrelated arguments in set theory are, in fact, concerned with just such a problem. It might therefore be of interest to study such relations for their own sake and to build up a partition calculus which might serve as a new and unifying principle in set theory. (Source Project Euclid.)
In the early 1950s, Rado introduced the partition calculus notation. For example
Ramsey's Theorem: For any \(n,m\lt \omega\text{,}\) one has \(\omega\rightarrow(\omega)^n_m\text{.}\)
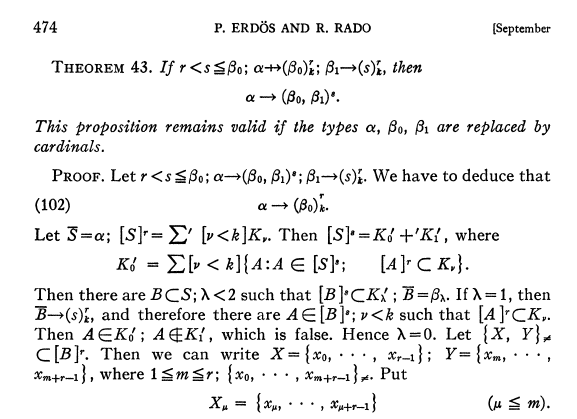
Example 4.3.6.
Rado Graph.
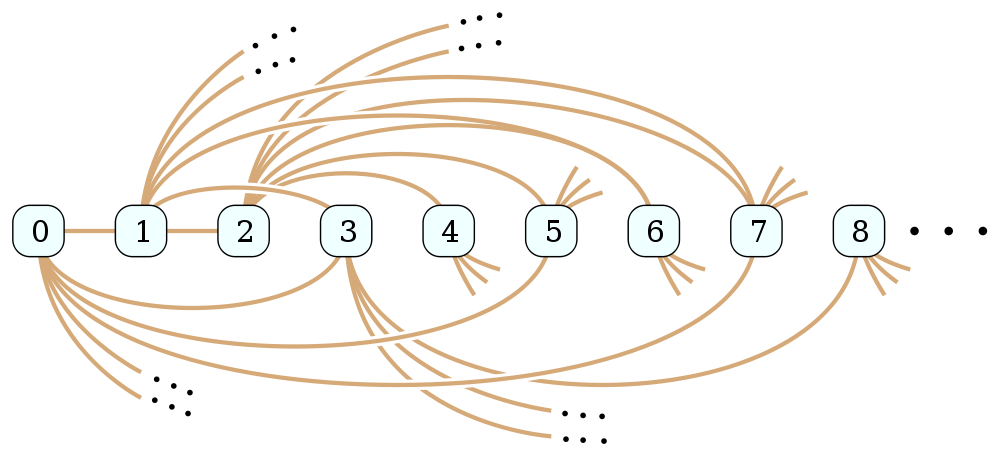
In 1964 Rado constructed the Rado graph by identifying the vertices of the graph with the natural numbers \(0, 1, 2, \ldots\text{.}\) An edge connects vertices \(x\) and \(y\) in the graph (with \(x \lt y\)) whenever the \(x\)th bit of the binary representation of \(y\) is nonzero. Thus, for instance, the neighbours of vertex 0 consist of all odd-numbered vertices, while the neighbours of vertex 1 consist of vertex 0 (the only vertex whose bit in the binary representation of 1 is nonzero) and all vertices with numbers congruent to \(2\) or \(3\) modulo \(4\text{.}\)
Resources.
For mored details see [7], pp. 304-308.
Mathematicians Begin to Tame Wild “Sunflower” Problem by Kevin Hartnett