Section 1.3 Frank Plumpton Ramsey
Philosophy must be of some use and we must take it seriously; it must clear our thoughts and so our actions. — Frank Plumpton Ramsey, 1903 — 1930.
Who was Frank Ramsey? British mathematician, economist, and philosopher.
Ramsey made contributions to:
epistemology; the branch of philosophy concerned with the nature and scope of knowledge
semantics; the study of relationships between signs and symbols and what they represent
logic; the study of the principles of reasoning
philosophy of science; the field of rigorous academic study that deals specifically with what science is, how it works, and the logic through which we build scientific knowledge
decision theory; decision theory in economics, psychology, philosophy, mathematics, and statistics is concerned with identifying the values, uncertainties and other issues relevant in a given decision, its rationality, and the resulting optimal decision.
metaphysics; the part of philosophy that is concerned with the basic causes and nature of things.
mathematics
statistics
probability
economics
Birth and Death. Frank Plumpton Ramsey was born on February 22, 1903, and died at the age of 26 on January 19, 1930. Ramsey suffered from a chronic and increasingly serious liver complaint, contracted jaundice after an operation and died at Guy's Hospital in London.
World in 1903
World in 1930
The first west–east transatlantic radio broadcast was made from the US to England
Mao Tse-tung writes A Single Spark Can Star a Prairie Fire
Bertrand Russell published “The Principles of Mathematics”
The first diesel engine automobile trip completed
Wright Brothers make the first flight
The first radar detection of planes
Pepsi Cola company forms
The world's first radiosonde is launched
Paavo Nurmi runs world record 6 mile 29:36.4
The Mersenne number \(2^{67}-1\) discovered
The Russian Social Democratic Labor Party splits into two groups; the Bolsheviks and Mensheviks
Child labor laws established in Belgium
The automobile electric starter patented
The first non-stop airplane flight from Europe to US
Nobel for physics awarded to Pierre and Marie Curie
Nazis gain 107 seats in German election
Frank's Family. Ramsey came from a distinguished Cambridge family. His father was a mathematician, and the President of Magdalene College. His brother, Michael Ramsey, became the Archbishop of Canterbury. His sister Bridget was a medical doctor, and his other sister Margaret was a Fellow of Lady Margaret Hall, Oxford.
In 1924, at the age of twenty-one, Ramsey himself got a Fellowship at King's College Cambridge, having graduated the year before as Cambridge's top mathematics student.
Michael Ramsey about his brother Frank:
He was interested in almost everything. He was immensely widely read in English literature; he was enjoying classics though he was on the verge of plunging into being a mathematical specialist; he was very interested in politics, and well-informed; he had got a political concern and a sort of left-wing caring-for-the-underdog kind of outlook about politics. I was aware that he was far cleverer than I was and knew much more, yet there was such a total lack of uppishness about him that we just conversed in a friendly way and he never made me feel inferior though I was so vastly below par intellectually, and that was the wonderful joy of it. (Source D.H. Mellor ).
Ramsey's Work - Two Examples.
Example 1.3.2.
Conditionals:
In “General Propositions and Causality” (1929) Frank Ramsey wrote:
When we deliberate about a possible action, we ask ourselves what will happen if we do this or that. If we give a definite answer of the form ‘If I do \(p\text{,}\) \(q\) will result’, this can be properly regarded as a material implication or disjunction ‘Either not \(p\text{,}\) or \(q\)’. But it differs, of course from any ordinary disjunction in that one of its members is not something of which we are trying to discover the truth, but something within our power to make true or false. Besides definite answers ‘If \(p\text{,}\) \(q\) will result’, we often get ones `If \(p\text{,}\) \(q\) might result or ‘\(q\) would probably result’. Here the degree of probability is not a degree of belief in ‘Not-\(p\) or \(q\)’, but a degree of belief in \(q\) given \(p\text{,}\) which it is evidently possible to have without a definite degree of belief in \(p\text{,}\) \(p\) not being an intellectual problem. And our conduct is largely determined by these degrees of hypothetical belief. (Source Stanford Encyclopedia of Philosophy).
For example, take:
\(p=\) Dr. J explains to his students that cheating on exams is bad for their academic growth.
and
\(q=\) Dr. J's students do not cheat on exams.
Observe that
Not- \(p =\) Dr. J does not explain to his students that cheating on exams is bad for their academic growth.
Recall that in the formal logic:
Suppose that Dr. J thinks: “If I do \(p\) then \(q\) will result.”
If this was an “ordinary” implication it would be equivalent to
“Dr. J does not explain to his students that cheating on exams is bad for their academic growth” OR “Dr. J's students do not cheat on exams.”
To paraphrase Ramsey's words: What Dr. J really thinks is not ‘If \(p\text{,}\) \(q\) will result’, but rather ‘If \(p\text{,}\) \(q\) might result’ or ‘\(q\) would probably result’. Here the degree of probability is not a degree of Dr. J's belief in ‘Not-\(p\) or q’ but a degree of Dr. J's belief in \(q\) given \(p\text{.}\)
Example 1.3.3.
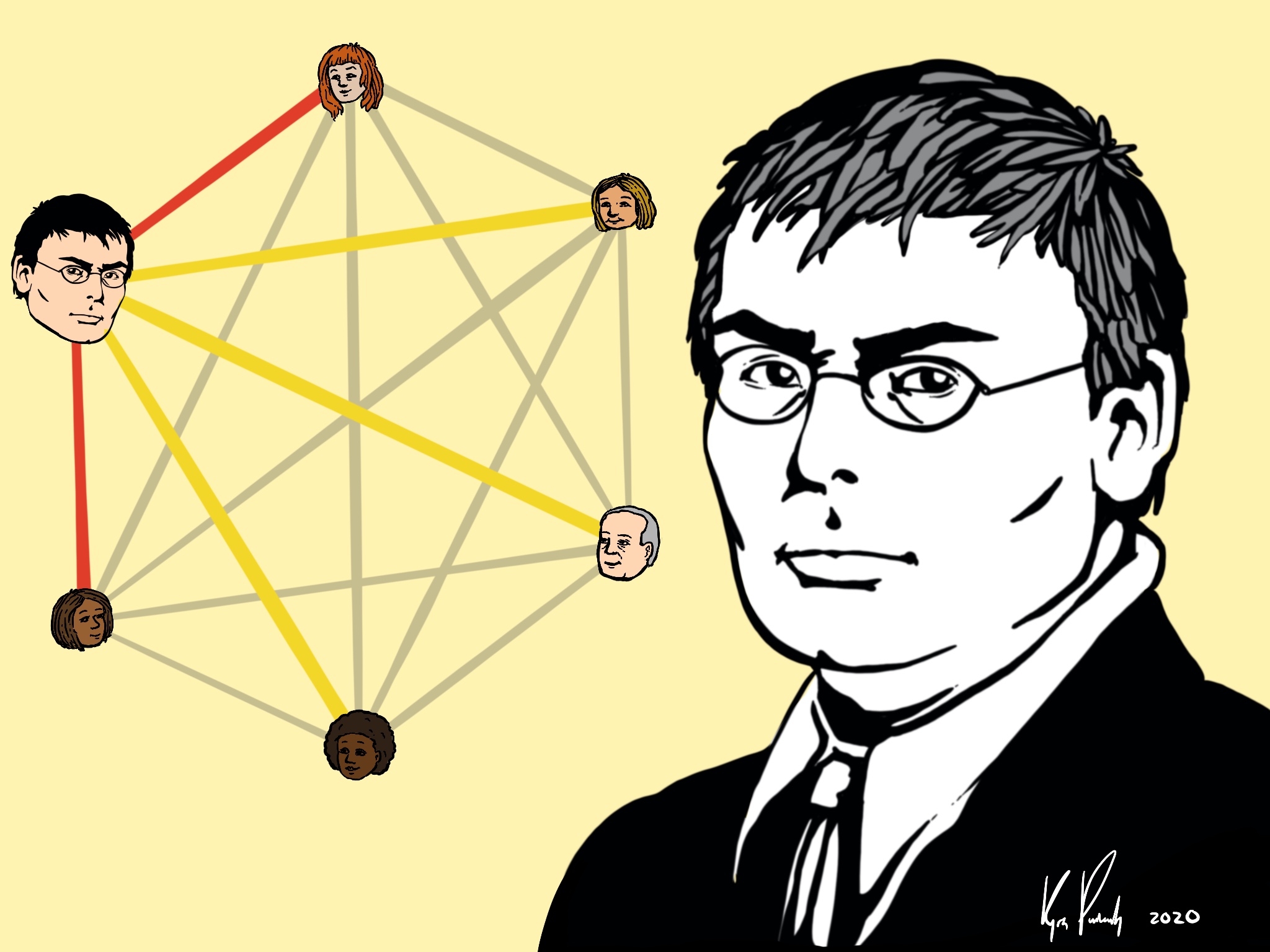
Ramsey's Theorem.
In On a Problem of Formal Logic, Proceedings of the London Mathematical Society, 1930 [6]:
Given any \(r\text{,}\) \(n\text{,}\) and \(\mu\) we can find an \(m_0\) such that, if \(m\geq m_0\) and the \(r\)- combinations of any \(\Gamma _m\) are divided in any manner into \(\mu\) mutually exclusive classes \(C_i\) \((i=1,2,\ldots ,\mu)\text{,}\) then \(\Gamma _m\) must contain a sub-class \(\Delta _n\) such that all the \(r\)-combinations of members of \(\Delta _n\) belong to the same \(C_i\text{.}\)
Ramsey Theory. Today Ramsey's Theorem is one of the cornerstones of Ramsey theory. Some other results that form the very base of Ramsey theory are Hilbert's Theorem (1892), Schur's Theorem (1916), and van der Waerden's Theorem (1927).
This clearly contradicts the statement by David Hugh Mellor [5] that
Frank P. Ramsey will be known to readers of the Journal of Graph Theory as the eponymous discoverer of Ramsey numbers and founder of Ramsey theory (…)
So, when did Ramsey theory become Ramsey theory?
Alexander Soifer [7] offers a detailed account of his own investigation about this question and concludes:
It seems that The Ramsey Theory has been shaping throughout the 1970s, and the central engine of this process was new results and two surveys by Graham and Rothschild. In 1980 the long life of the name was assured when it appeared as the title of the book Ramsey Theory by Graham, Rothschild, and Spencer.
Joel Spencer [8] gives us the birthplace of Ramsey theory:
In my opinion, Ramsey theory was born, after a long and healthy embryonic stage, at the Combinatorial Conference at Balatonfüred, Hungary, 1973.
Who was the father? In the preface to the first edition of the book [2] the authors, Graham, Rothschild, and Spencer, attribute Paul Erdős as one
who can rightfully be considered the father of modern Ramsey theory.
Resources.